Imitating Language via Scalable Inverse Reinforcement Learning

The majority of language model training builds on imitation learning. It covers pretraining, supervised fine-tuning, and affects the starting conditions for reinforcement learning from human feedback (RLHF). The simplicity and scalability of maximum likelihood estimation (MLE) for next token prediction led to its role as predominant paradigm. However, the broader field of imitation learning can more effectively utilize the sequential structure underlying autoregressive generation. We focus on investigating the inverse reinforcement learning (IRL) perspective to imitation, extracting rewards and directly optimizing sequences instead of individual token likelihoods and evaluate its benefits for fine-tuning large language models. We provide a new angle, reformulating inverse soft-Q-learning as a temporal difference regularized extension of MLE. This creates a principled connection between MLE and IRL and allows trading off added complexity with increased performance and diversity of generations in the supervised fine-tuning (SFT) setting. We find clear advantages for IRL-based imitation, in particular for retaining diversity while maximizing task performance, rendering IRL a strong alternative on fixed SFT datasets even without online data generation. Our analysis of IRL-extracted reward functions further indicates benefits for more robust reward functions via tighter integration of supervised and preference-based LLM post-training.

RecurrentGemma: Moving Past Transformers for Efficient Open Language Models

We introduce RecurrentGemma, an open language model which uses Google's novel Griffin architecture. Griffin combines linear recurrences with local attention to achieve excellent performance on language. It has a fixed-sized state, which reduces memory use and enables efficient inference on long sequences. We provide a pre-trained model with 2B non-embedding parameters, and an instruction tuned variant. Both models achieve comparable performance to Gemma-2B despite being trained on fewer tokens.

Griffin: Mixing Gated Linear Recurrences with Local Attention for Efficient Language Models

Recurrent neural networks (RNNs) have fast inference and scale efficiently on long sequences, but they are difficult to train and hard to scale. We propose Hawk, an RNN with gated linear recurrences, and Griffin, a hybrid model that mixes gated linear recurrences with local attention. Hawk exceeds the reported performance of Mamba on downstream tasks, while Griffin matches the performance of Llama-2 despite being trained on over 6 times fewer tokens. We also show that Griffin can extrapolate on sequences significantly longer than those seen during training. Our models match the hardware efficiency of Transformers during training, and during inference they have lower latency and significantly higher throughput. We scale Griffin up to 14B parameters, and explain how to shard our models for efficient distributed training.

Gemini: A Family of Highly Capable Multimodal Models

This report introduces a new family of multimodal models, Gemini, that exhibit remarkable capabilities across image, audio, video, and text understanding. The Gemini family consists of Ultra, Pro, and Nano sizes, suitable for applications ranging from complex reasoning tasks to on-device memory-constrained use-cases. Evaluation on a broad range of benchmarks shows that our most-capable Gemini Ultra model advances the state of the art in 30 of 32 of these benchmarks - notably being the first model to achieve human-expert performance on the well-studied exam benchmark MMLU, and improving the state of the art in every one of the 20 multimodal benchmarks we examined. We believe that the new capabilities of Gemini models in cross-modal reasoning and language understanding will enable a wide variety of use cases and we discuss our approach toward deploying them responsibly to users.

APART: Diverse Skill Discovery using All Pairs with Ascending Reward and DropouT

We study diverse skill discovery in reward-free environments, aiming to discover all possible skills in simple grid-world environments where prior methods have struggled to succeed. This problem is formulated as mutual training of skills using an intrinsic reward and a discriminator trained to predict a skill given its trajectory. Our initial solution replaces the standard one-vs-all (softmax) discriminator with a one-vs-one (all pairs) discriminator and combines it with a novel intrinsic reward function and a dropout regularization technique. The combined approach is named APART: Diverse Skill Discovery using All Pairs with Ascending Reward and Dropout. We demonstrate that APART discovers all the possible skills in grid worlds with remarkably fewer samples than previous works. Motivated by the empirical success of APART, we further investigate an even simpler algorithm that achieves maximum skills by altering VIC, rescaling its intrinsic reward, and tuning the temperature of its softmax discriminator. We believe our findings shed light on the crucial factors underlying success of skill discovery algorithms in reinforcement learning.

Rapid training of deep neural networks without skip connections or normalization layers using Deep Kernel Shaping

Using an extended and formalized version of the Q/C map analysis of Poole et al. (2016), along with Neural Tangent Kernel theory, we identify the main pathologies present in deep networks that prevent them from training fast and generalizing to unseen data, and show how these can be avoided by carefully controlling the "shape" of the network's initialization-time kernel function. We then develop a method called Deep Kernel Shaping (DKS), which accomplishes this using a combination of precise parameter initialization, activation function transformations, and small architectural tweaks, all of which preserve the model class. In our experiments we show that DKS enables SGD training of residual networks without normalization layers on Imagenet and CIFAR-10 classification tasks at speeds comparable to standard ResNetV2 and Wide-ResNet models, with only a small decrease in generalization performance. And when using K-FAC as the optimizer, we achieve similar results for networks without skip connections. Our results apply for a large variety of activation functions, including those which traditionally perform very badly, such as the logistic sigmoid. In addition to DKS, we contribute a detailed analysis of skip connections, normalization layers, special activation functions like RELU and SELU, and various initialization schemes, explaining their effectiveness as alternative (and ultimately incomplete) ways of "shaping" the network's initialization-time kernel.

Reward is enough for convex MDPs

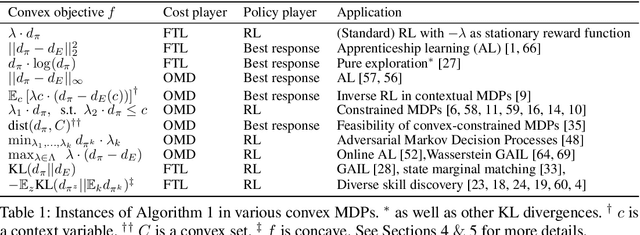
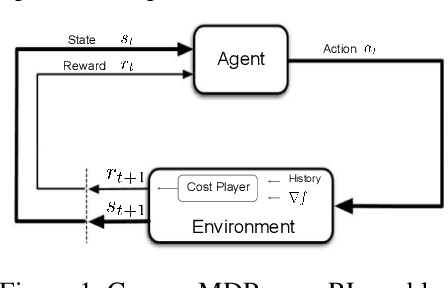
Maximising a cumulative reward function that is Markov and stationary, i.e., defined over state-action pairs and independent of time, is sufficient to capture many kinds of goals in a Markov Decision Process (MDP) based on the Reinforcement Learning (RL) problem formulation. However, not all goals can be captured in this manner. Specifically, it is easy to see that Convex MDPs in which goals are expressed as convex functions of stationary distributions cannot, in general, be formulated in this manner. In this paper, we reformulate the convex MDP problem as a min-max game between the policy and cost (negative reward) players using Fenchel duality and propose a meta-algorithm for solving it. We show that the average of the policies produced by an RL agent that maximizes the non-stationary reward produced by the cost player converges to an optimal solution to the convex MDP. Finally, we show that the meta-algorithm unifies several disparate branches of reinforcement learning algorithms in the literature, such as apprenticeship learning, variational intrinsic control, constrained MDPs, and pure exploration into a single framework.

Behavior Priors for Efficient Reinforcement Learning

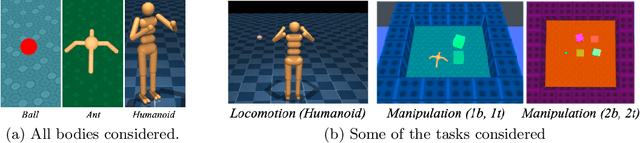
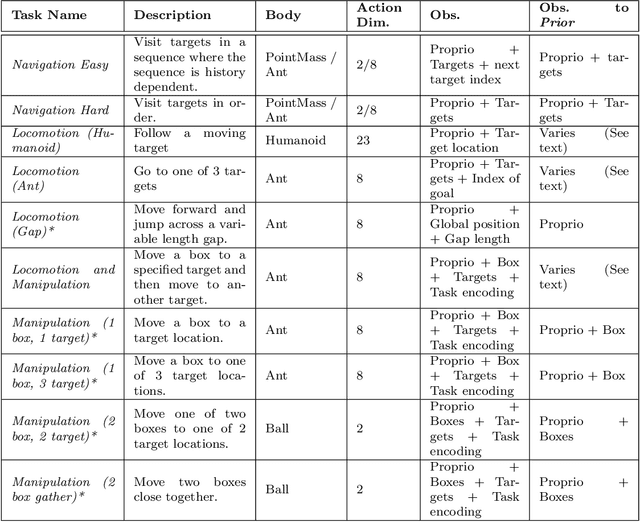
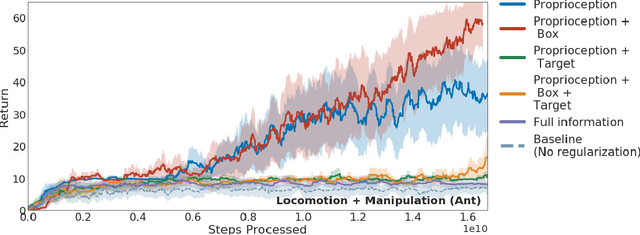
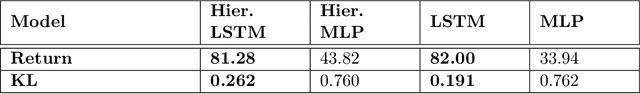
As we deploy reinforcement learning agents to solve increasingly challenging problems, methods that allow us to inject prior knowledge about the structure of the world and effective solution strategies becomes increasingly important. In this work we consider how information and architectural constraints can be combined with ideas from the probabilistic modeling literature to learn behavior priors that capture the common movement and interaction patterns that are shared across a set of related tasks or contexts. For example the day-to day behavior of humans comprises distinctive locomotion and manipulation patterns that recur across many different situations and goals. We discuss how such behavior patterns can be captured using probabilistic trajectory models and how these can be integrated effectively into reinforcement learning schemes, e.g.\ to facilitate multi-task and transfer learning. We then extend these ideas to latent variable models and consider a formulation to learn hierarchical priors that capture different aspects of the behavior in reusable modules. We discuss how such latent variable formulations connect to related work on hierarchical reinforcement learning (HRL) and mutual information and curiosity based objectives, thereby offering an alternative perspective on existing ideas. We demonstrate the effectiveness of our framework by applying it to a range of simulated continuous control domains.

Importance Weighted Policy Learning and Adaption

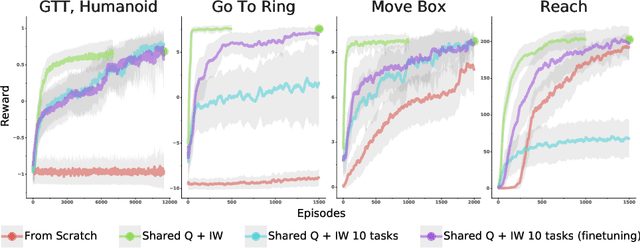
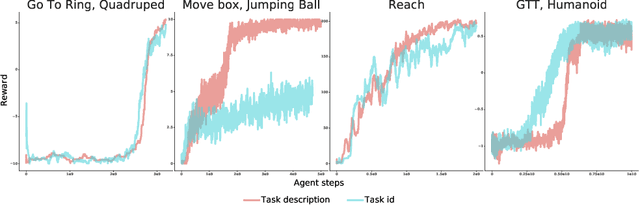
The ability to exploit prior experience to solve novel problems rapidly is a hallmark of biological learning systems and of great practical importance for artificial ones. In the meta reinforcement learning literature much recent work has focused on the problem of optimizing the learning process itself. In this paper we study a complementary approach which is conceptually simple, general, modular and built on top of recent improvements in off-policy learning. The framework is inspired by ideas from the probabilistic inference literature and combines robust off-policy learning with a behavior prior, or default behavior that constrains the space of solutions and serves as a bias for exploration; as well as a representation for the value function, both of which are easily learned from a number of training tasks in a multi-task scenario. Our approach achieves competitive adaptation performance on hold-out tasks compared to meta reinforcement learning baselines and can scale to complex sparse-reward scenarios.

Information asymmetry in KL-regularized RL

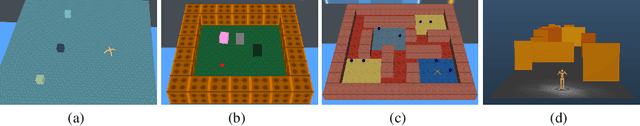
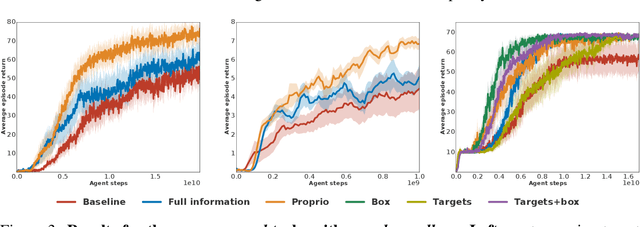
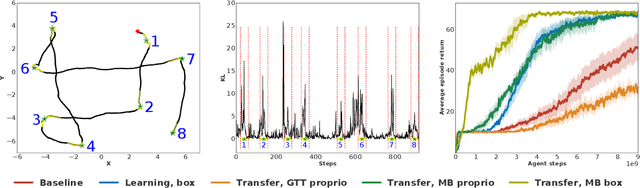
Many real world tasks exhibit rich structure that is repeated across different parts of the state space or in time. In this work we study the possibility of leveraging such repeated structure to speed up and regularize learning. We start from the KL regularized expected reward objective which introduces an additional component, a default policy. Instead of relying on a fixed default policy, we learn it from data. But crucially, we restrict the amount of information the default policy receives, forcing it to learn reusable behaviors that help the policy learn faster. We formalize this strategy and discuss connections to information bottleneck approaches and to the variational EM algorithm. We present empirical results in both discrete and continuous action domains and demonstrate that, for certain tasks, learning a default policy alongside the policy can significantly speed up and improve learning.