Evaluating Podcast Recommendations with Profile-Aware LLM-as-a-Judge

Evaluating personalized recommendations remains a central challenge, especially in long-form audio domains like podcasts, where traditional offline metrics suffer from exposure bias and online methods such as A/B testing are costly and operationally constrained. In this paper, we propose a novel framework that leverages Large Language Models (LLMs) as offline judges to assess the quality of podcast recommendations in a scalable and interpretable manner. Our two-stage profile-aware approach first constructs natural-language user profiles distilled from 90 days of listening history. These profiles summarize both topical interests and behavioral patterns, serving as compact, interpretable representations of user preferences. Rather than prompting the LLM with raw data, we use these profiles to provide high-level, semantically rich context-enabling the LLM to reason more effectively about alignment between a user's interests and recommended episodes. This reduces input complexity and improves interpretability. The LLM is then prompted to deliver fine-grained pointwise and pairwise judgments based on the profile-episode match. In a controlled study with 47 participants, our profile-aware judge matched human judgments with high fidelity and outperformed or matched a variant using raw listening histories. The framework enables efficient, profile-aware evaluation for iterative testing and model selection in recommender systems.

Text2Tracks: Prompt-based Music Recommendation via Generative Retrieval

In recent years, Large Language Models (LLMs) have enabled users to provide highly specific music recommendation requests using natural language prompts (e.g. "Can you recommend some old classics for slow dancing?"). In this setup, the recommended tracks are predicted by the LLM in an autoregressive way, i.e. the LLM generates the track titles one token at a time. While intuitive, this approach has several limitation. First, it is based on a general purpose tokenization that is optimized for words rather than for track titles. Second, it necessitates an additional entity resolution layer that matches the track title to the actual track identifier. Third, the number of decoding steps scales linearly with the length of the track title, slowing down inference. In this paper, we propose to address the task of prompt-based music recommendation as a generative retrieval task. Within this setting, we introduce novel, effective, and efficient representations of track identifiers that significantly outperform commonly used strategies. We introduce Text2Tracks, a generative retrieval model that learns a mapping from a user's music recommendation prompt to the relevant track IDs directly. Through an offline evaluation on a dataset of playlists with language inputs, we find that (1) the strategy to create IDs for music tracks is the most important factor for the effectiveness of Text2Tracks and semantic IDs significantly outperform commonly used strategies that rely on song titles as identifiers (2) provided with the right choice of track identifiers, Text2Tracks outperforms sparse and dense retrieval solutions trained to retrieve tracks from language prompts.

Policy-as-Prompt: Rethinking Content Moderation in the Age of Large Language Models

Content moderation plays a critical role in shaping safe and inclusive online environments, balancing platform standards, user expectations, and regulatory frameworks. Traditionally, this process involves operationalising policies into guidelines, which are then used by downstream human moderators for enforcement, or to further annotate datasets for training machine learning moderation models. However, recent advancements in large language models (LLMs) are transforming this landscape. These models can now interpret policies directly as textual inputs, eliminating the need for extensive data curation. This approach offers unprecedented flexibility, as moderation can be dynamically adjusted through natural language interactions. This paradigm shift raises important questions about how policies are operationalised and the implications for content moderation practices. In this paper, we formalise the emerging policy-as-prompt framework and identify five key challenges across four domains: Technical Implementation (1. translating policy to prompts, 2. sensitivity to prompt structure and formatting), Sociotechnical (3. the risk of technological determinism in policy formation), Organisational (4. evolving roles between policy and machine learning teams), and Governance (5. model governance and accountability). Through analysing these challenges across technical, sociotechnical, organisational, and governance dimensions, we discuss potential mitigation approaches. This research provides actionable insights for practitioners and lays the groundwork for future exploration of scalable and adaptive content moderation systems in digital ecosystems.

Generating Query Recommendations via LLMs

Query recommendation systems are ubiquitous in modern search engines, assisting users in producing effective queries to meet their information needs. However, these systems require a large amount of data to produce good recommendations, such as a large collection of documents to index and query logs. In particular, query logs and user data are not available in cold start scenarios. Query logs are expensive to collect and maintain and require complex and time-consuming cascading pipelines for creating, combining, and ranking recommendations. To address these issues, we frame the query recommendation problem as a generative task, proposing a novel approach called Generative Query Recommendation (GQR). GQR uses an LLM as its foundation and does not require to be trained or fine-tuned to tackle the query recommendation problem. We design a prompt that enables the LLM to understand the specific recommendation task, even using a single example. We then improved our system by proposing a version that exploits query logs called Retriever-Augmented GQR (RA-GQR). RA-GQr dynamically composes its prompt by retrieving similar queries from query logs. GQR approaches reuses a pre-existing neural architecture resulting in a simpler and more ready-to-market approach, even in a cold start scenario. Our proposed GQR obtains state-of-the-art performance in terms of NDCG@10 and clarity score against two commercial search engines and the previous state-of-the-art approach on the Robust04 and ClueWeb09B collections, improving on average the NDCG@10 performance up to ~4% on Robust04 and ClueWeb09B w.r.t the previous best competitor. RA-GQR further improve the NDCG@10 obtaining an increase of ~11%, ~6\% on Robust04 and ClueWeb09B w.r.t the best competitor. Furthermore, our system obtained ~59% of user preferences in a blind user study, proving that our method produces the most engaging queries.

Towards Graph Foundation Models for Personalization

In the realm of personalization, integrating diverse information sources such as consumption signals and content-based representations is becoming increasingly critical to build state-of-the-art solutions. In this regard, two of the biggest trends in research around this subject are Graph Neural Networks (GNNs) and Foundation Models (FMs). While GNNs emerged as a popular solution in industry for powering personalization at scale, FMs have only recently caught attention for their promising performance in personalization tasks like ranking and retrieval. In this paper, we present a graph-based foundation modeling approach tailored to personalization. Central to this approach is a Heterogeneous GNN (HGNN) designed to capture multi-hop content and consumption relationships across a range of recommendable item types. To ensure the generality required from a Foundation Model, we employ a Large Language Model (LLM) text-based featurization of nodes that accommodates all item types, and construct the graph using co-interaction signals, which inherently transcend content specificity. To facilitate practical generalization, we further couple the HGNN with an adaptation mechanism based on a two-tower (2T) architecture, which also operates agnostically to content type. This multi-stage approach ensures high scalability; while the HGNN produces general purpose embeddings, the 2T component models in a continuous space the sheer size of user-item interaction data. Our comprehensive approach has been rigorously tested and proven effective in delivering recommendations across a diverse array of products within a real-world, industrial audio streaming platform.

Personalized Audiobook Recommendations at Spotify Through Graph Neural Networks

In the ever-evolving digital audio landscape, Spotify, well-known for its music and talk content, has recently introduced audiobooks to its vast user base. While promising, this move presents significant challenges for personalized recommendations. Unlike music and podcasts, audiobooks, initially available for a fee, cannot be easily skimmed before purchase, posing higher stakes for the relevance of recommendations. Furthermore, introducing a new content type into an existing platform confronts extreme data sparsity, as most users are unfamiliar with this new content type. Lastly, recommending content to millions of users requires the model to react fast and be scalable. To address these challenges, we leverage podcast and music user preferences and introduce 2T-HGNN, a scalable recommendation system comprising Heterogeneous Graph Neural Networks (HGNNs) and a Two Tower (2T) model. This novel approach uncovers nuanced item relationships while ensuring low latency and complexity. We decouple users from the HGNN graph and propose an innovative multi-link neighbor sampler. These choices, together with the 2T component, significantly reduce the complexity of the HGNN model. Empirical evaluations involving millions of users show significant improvement in the quality of personalized recommendations, resulting in a +46% increase in new audiobooks start rate and a +23% boost in streaming rates. Intriguingly, our model's impact extends beyond audiobooks, benefiting established products like podcasts.

Fast Adaptation with Linearized Neural Networks

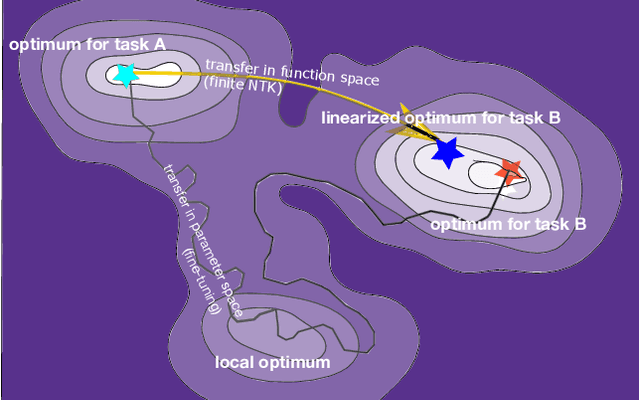
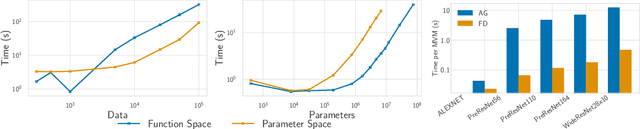
The inductive biases of trained neural networks are difficult to understand and, consequently, to adapt to new settings. We study the inductive biases of linearizations of neural networks, which we show to be surprisingly good summaries of the full network functions. Inspired by this finding, we propose a technique for embedding these inductive biases into Gaussian processes through a kernel designed from the Jacobian of the network. In this setting, domain adaptation takes the form of interpretable posterior inference, with accompanying uncertainty estimation. This inference is analytic and free of local optima issues found in standard techniques such as fine-tuning neural network weights to a new task. We develop significant computational speed-ups based on matrix multiplies, including a novel implementation for scalable Fisher vector products. Our experiments on both image classification and regression demonstrate the promise and convenience of this framework for transfer learning, compared to neural network fine-tuning. Code is available at https://github.com/amzn/xfer/tree/master/finite_ntk.

Tomographic Auto-Encoder: Unsupervised Bayesian Recovery of Corrupted Data

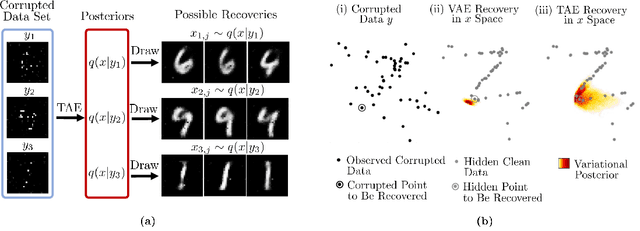
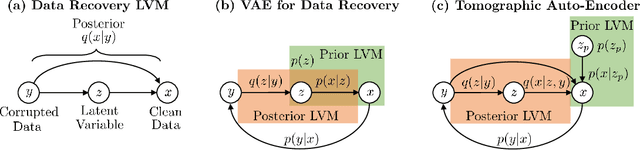
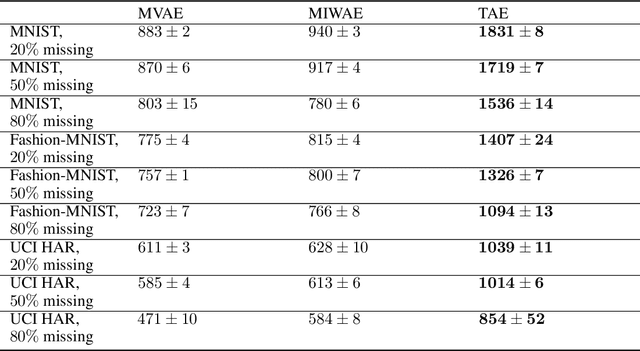
We propose a new probabilistic method for unsupervised recovery of corrupted data. Given a large ensemble of degraded samples, our method recovers accurate posteriors of clean values, allowing the exploration of the manifold of possible reconstructed data and hence characterising the underlying uncertainty. In this setting, direct application of classical variational methods often gives rise to collapsed densities that do not adequately explore the solution space. Instead, we derive our novel reduced entropy condition approximate inference method that results in rich posteriors. We test our model in a data recovery task under the common setting of missing values and noise, demonstrating superior performance to existing variational methods for imputation and de-noising with different real data sets. We further show higher classification accuracy after imputation, proving the advantage of propagating uncertainty to downstream tasks with our model.

Empirical Bayes Transductive Meta-Learning with Synthetic Gradients

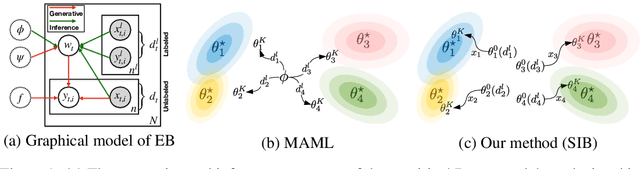
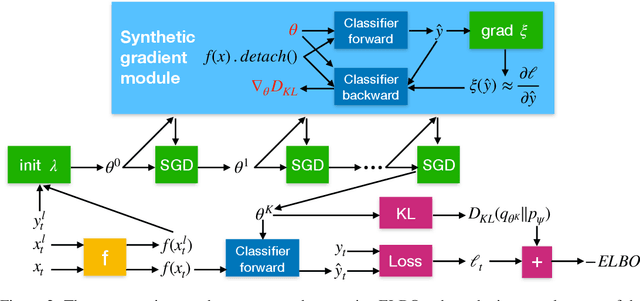
We propose a meta-learning approach that learns from multiple tasks in a transductive setting, by leveraging the unlabeled query set in addition to the support set to generate a more powerful model for each task. To develop our framework, we revisit the empirical Bayes formulation for multi-task learning. The evidence lower bound of the marginal log-likelihood of empirical Bayes decomposes as a sum of local KL divergences between the variational posterior and the true posterior on the query set of each task. We derive a novel amortized variational inference that couples all the variational posteriors via a meta-model, which consists of a synthetic gradient network and an initialization network. Each variational posterior is derived from synthetic gradient descent to approximate the true posterior on the query set, although where we do not have access to the true gradient. Our results on the Mini-ImageNet and CIFAR-FS benchmarks for episodic few-shot classification outperform previous state-of-the-art methods. Besides, we conduct two zero-shot learning experiments to further explore the potential of the synthetic gradient.

Online Constrained Model-based Reinforcement Learning

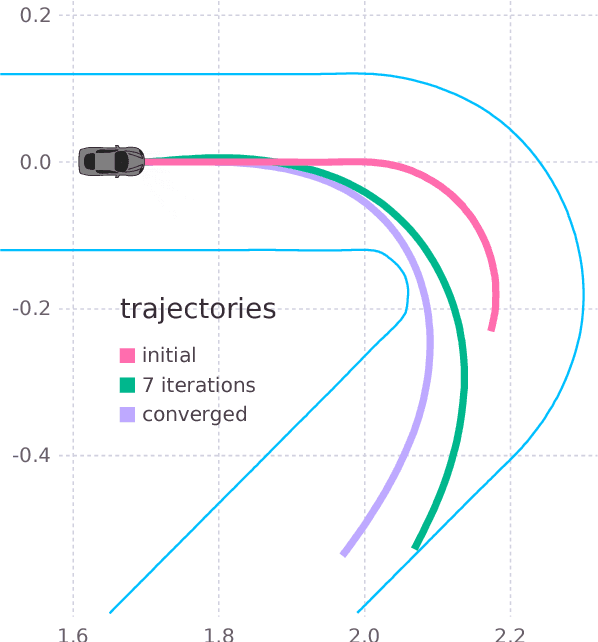
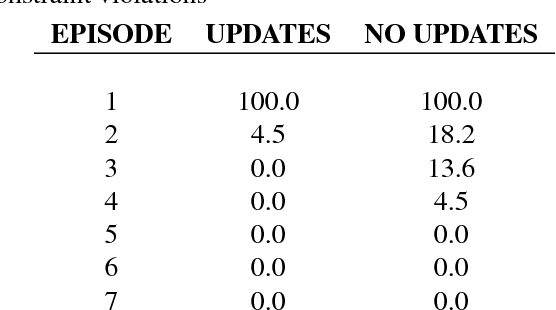
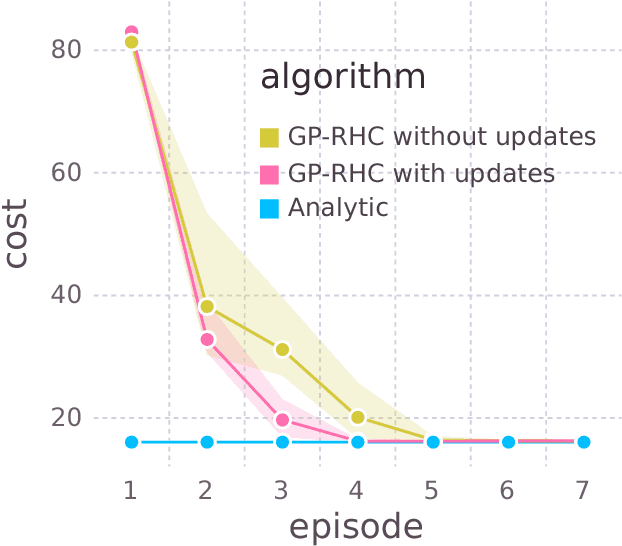
Applying reinforcement learning to robotic systems poses a number of challenging problems. A key requirement is the ability to handle continuous state and action spaces while remaining within a limited time and resource budget. Additionally, for safe operation, the system must make robust decisions under hard constraints. To address these challenges, we propose a model based approach that combines Gaussian Process regression and Receding Horizon Control. Using sparse spectrum Gaussian Processes, we extend previous work by updating the dynamics model incrementally from a stream of sensory data. This results in an agent that can learn and plan in real-time under non-linear constraints. We test our approach on a cart pole swing-up environment and demonstrate the benefits of online learning on an autonomous racing task. The environment's dynamics are learned from limited training data and can be reused in new task instances without retraining.