Assisted Physical Interaction: Autonomous Aerial Robots with Neural Network Detection, Navigation, and Safety Layers

The paper introduces a novel framework for safe and autonomous aerial physical interaction in industrial settings. It comprises two main components: a neural network-based target detection system enhanced with edge computing for reduced onboard computational load, and a control barrier function (CBF)-based controller for safe and precise maneuvering. The target detection system is trained on a dataset under challenging visual conditions and evaluated for accuracy across various unseen data with changing lighting conditions. Depth features are utilized for target pose estimation, with the entire detection framework offloaded into low-latency edge computing. The CBF-based controller enables the UAV to converge safely to the target for precise contact. Simulated evaluations of both the controller and target detection are presented, alongside an analysis of real-world detection performance.

Design of a Flexible Robot Arm for Safe Aerial Physical Interaction

This paper introduces a novel compliant mechanism combining lightweight and energy dissipation for aerial physical interaction. Weighting 400~g at take-off, the mechanism is actuated in the forward body direction, enabling precise position control for force interaction and various other aerial manipulation tasks. The robotic arm, structured as a closed-loop kinematic chain, employs two deported servomotors. Each joint is actuated with a single tendon for active motion control in compression of the arm at the end-effector. Its elasto-mechanical design reduces weight and provides flexibility, allowing passive-compliant interactions without impacting the motors' integrity. Notably, the arm's damping can be adjusted based on the proposed inner frictional bulges. Experimental applications showcase the aerial system performance in both free-flight and physical interaction. The presented work may open safer applications for \ac{MAV} in real environments subject to perturbations during interaction.

Design and Control of an Omnidirectional Aerial Robot with a Miniaturized Haptic Joystick for Physical Interaction

Fully actuated aerial robot proved their superiority for Aerial Physical Interaction (APhI) over the past years. This work proposes a minimal setup for aerial telemanipulation, enhancing accessibility of these technologies. The design and the control of a 6-DoF joystick with 4-DoF haptic feedback is detailed. It is the first haptic device with standard Remote Controller (RC) form factor for APhI. By miniaturizing haptic device, it enhances RC with the sense of touch, increasing physical awareness. The goal is to give operators an extra sense, other than vision and sound, to help to perform safe APhI. To the best of the authors knowledge, this is the first teleoperation system able to decouple each single axis input command. On the omnidirectional quadrotor, by reducing the number of components with a new design, we aim a simplified maintenance, and improved force and thrust to weight ratio. Open-sourced physic based simulation and successful preliminary flight tests highlighted the tool as promising for future APhI applications.

AERIAL-CORE: AI-Powered Aerial Robots for Inspection and Maintenance of Electrical Power Infrastructures

Large-scale infrastructures are prone to deterioration due to age, environmental influences, and heavy usage. Ensuring their safety through regular inspections and maintenance is crucial to prevent incidents that can significantly affect public safety and the environment. This is especially pertinent in the context of electrical power networks, which, while essential for energy provision, can also be sources of forest fires. Intelligent drones have the potential to revolutionize inspection and maintenance, eliminating the risks for human operators, increasing productivity, reducing inspection time, and improving data collection quality. However, most of the current methods and technologies in aerial robotics have been trialed primarily in indoor testbeds or outdoor settings under strictly controlled conditions, always within the line of sight of human operators. Additionally, these methods and technologies have typically been evaluated in isolation, lacking comprehensive integration. This paper introduces the first autonomous system that combines various innovative aerial robots. This system is designed for extended-range inspections beyond the visual line of sight, features aerial manipulators for maintenance tasks, and includes support mechanisms for human operators working at elevated heights. The paper further discusses the successful validation of this system on numerous electrical power lines, with aerial robots executing flights over 10 kilometers away from their ground control stations.

Finite-time disturbance reconstruction and robust fractional-order controller design for hybrid port-Hamiltonian dynamics of biped robots

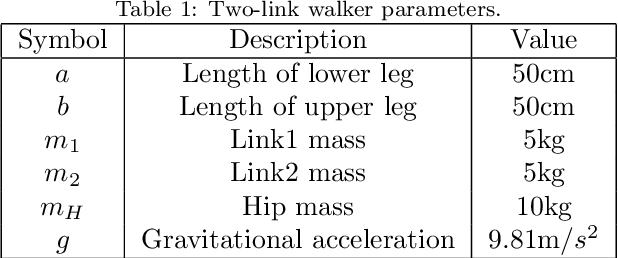
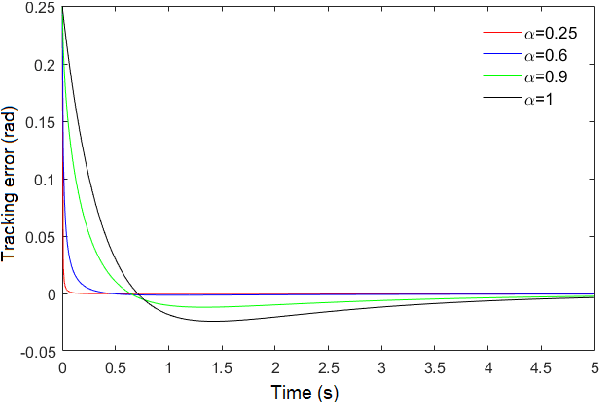
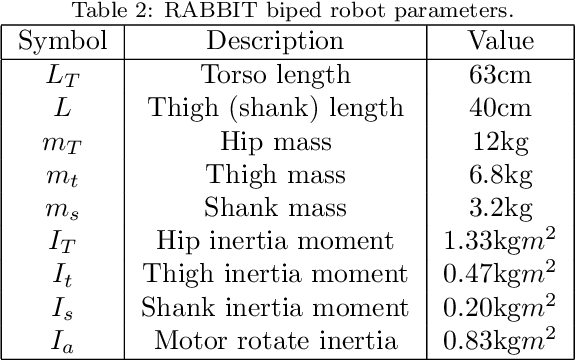
In this paper, disturbance reconstruction and robust trajectory tracking control of biped robots with hybrid dynamics in the port-Hamiltonian form is investigated. A new type of Hamiltonian function is introduced, which ensures the finite-time stability of the closed-loop system. The proposed control system consists of two loops: an inner and an outer loop. A fractional proportional-integral-derivative filter is used to achieve finite-time convergence for position tracking errors at the outer loop. A fractional-order sliding mode controller acts as a centralized controller at the inner-loop, ensuring the finite-time stability of the velocity tracking error. In this loop, the undesired effects of unknown external disturbance and parameter uncertainties are compensated using estimators. Two disturbance estimators are envisioned. The former is designed using fractional calculus. The latter is an adaptive estimator, and it is constructed using the general dynamic of biped robots. Stability analysis shows that the closed-loop system is finite-time stable in both contact-less and impact phases. Simulation studies on two types of biped robots (i.e., two-link walker and RABBIT biped robot) demonstrate the proposed controller's tracking performance and disturbance rejection capability.

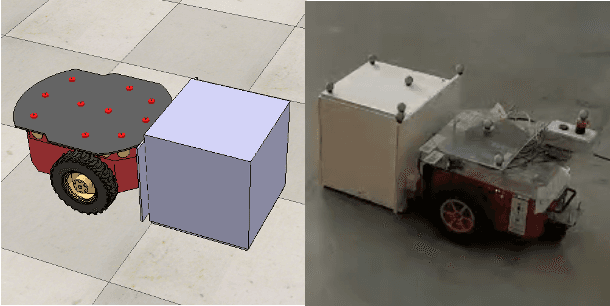
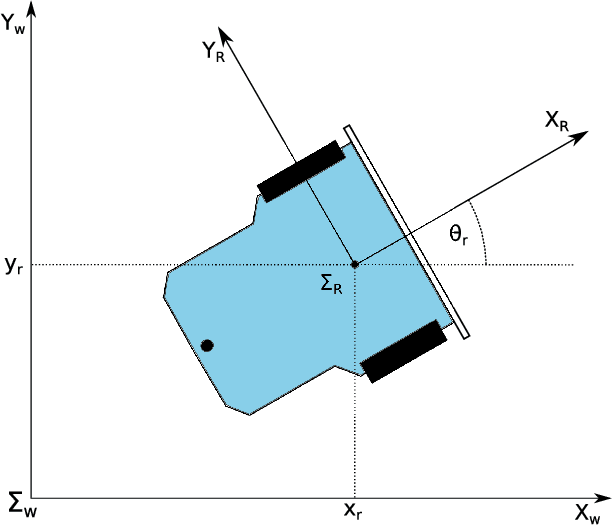
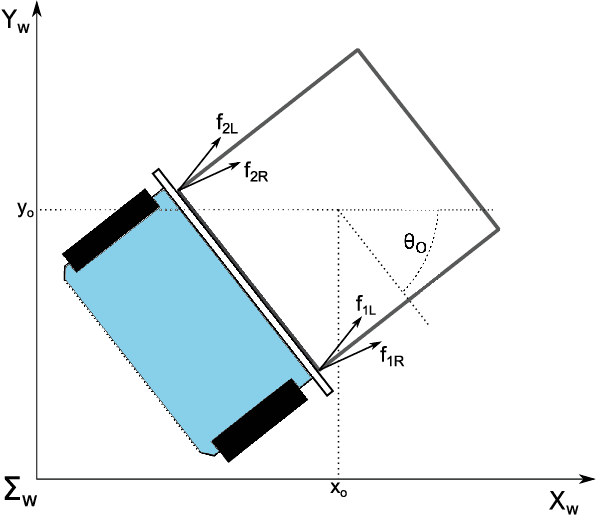
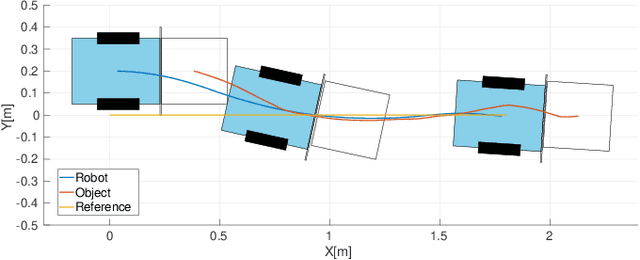
Linear Time-Varying MPC for Nonprehensile Object Manipulation with a Nonholonomic Mobile Robot

This paper proposes a technique to manipulate an object with a nonholonomic mobile robot by pushing, which is a nonprehensile manipulation motion primitive. Such a primitive involves unilateral constraints associated with the friction between the robot and the manipulated object. Violating this constraint produces the slippage of the object during the manipulation, preventing the correct achievement of the task. A linear time-varying model predictive control is designed to include the unilateral constraint within the control action properly. The approach is verified in a dynamic simulation environment through a Pioneer 3-DX wheeled robot executing the pushing manipulation of a package.