Meijia Shao
Distribution-Free Matrix Prediction Under Arbitrary Missing Pattern
May 19, 2023Abstract:This paper studies the open problem of conformalized entry prediction in a row/column-exchangeable matrix. The matrix setting presents novel and unique challenges, but there exists little work on this interesting topic. We meticulously define the problem, differentiate it from closely related problems, and rigorously delineate the boundary between achievable and impossible goals. We then propose two practical algorithms. The first method provides a fast emulation of the full conformal prediction, while the second method leverages the technique of algorithmic stability for acceleration. Both methods are computationally efficient and can effectively safeguard coverage validity in presence of arbitrary missing pattern. Further, we quantify the impact of missingness on prediction accuracy and establish fundamental limit results. Empirical evidence from synthetic and real-world data sets corroborates the superior performance of our proposed methods.
Higher-order accurate two-sample network inference and network hashing
Aug 16, 2022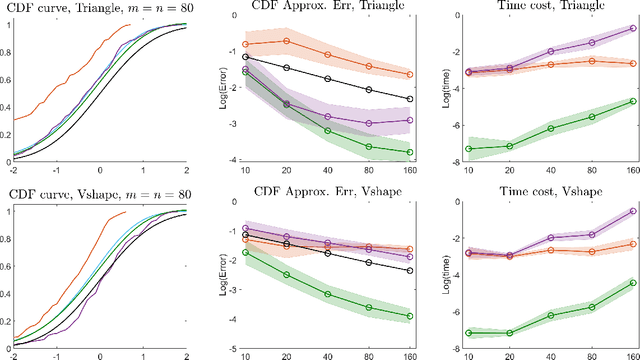


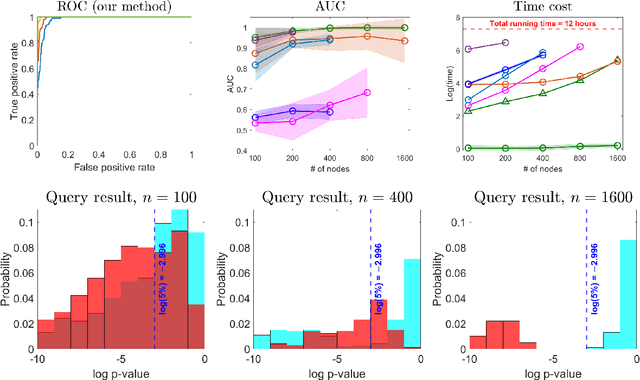
Abstract:Two-sample hypothesis testing for comparing two networks is an important yet difficult problem. Major challenges include: potentially different sizes and sparsity levels; non-repeated observations of adjacency matrices; computational scalability; and theoretical investigations, especially on finite-sample accuracy and minimax optimality. In this article, we propose the first provably higher-order accurate two-sample inference method by comparing network moments. Our method extends the classical two-sample t-test to the network setting. We make weak modeling assumptions and can effectively handle networks of different sizes and sparsity levels. We establish strong finite-sample theoretical guarantees, including rate-optimality properties. Our method is easy to implement and computes fast. We also devise a novel nonparametric framework of offline hashing and fast querying particularly effective for maintaining and querying very large network databases. We demonstrate the effectiveness of our method by comprehensive simulations. We apply our method to two real-world data sets and discover interesting novel structures.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge