CoT is Not the Chain of Truth: An Empirical Internal Analysis of Reasoning LLMs for Fake News Generation

From generating headlines to fabricating news, the Large Language Models (LLMs) are typically assessed by their final outputs, under the safety assumption that a refusal response signifies safe reasoning throughout the entire process. Challenging this assumption, our study reveals that during fake news generation, even when a model rejects a harmful request, its Chain-of-Thought (CoT) reasoning may still internally contain and propagate unsafe narratives. To analyze this phenomenon, we introduce a unified safety-analysis framework that systematically deconstructs CoT generation across model layers and evaluates the role of individual attention heads through Jacobian-based spectral metrics. Within this framework, we introduce three interpretable measures: stability, geometry, and energy to quantify how specific attention heads respond or embed deceptive reasoning patterns. Extensive experiments on multiple reasoning-oriented LLMs show that the generation risk rise significantly when the thinking mode is activated, where the critical routing decisions concentrated in only a few contiguous mid-depth layers. By precisely identifying the attention heads responsible for this divergence, our work challenges the assumption that refusal implies safety and provides a new understanding perspective for mitigating latent reasoning risks.

AFD-STA: Adaptive Filtering Denoising with Spatiotemporal Attention for Chaotic System Prediction

This paper presents AFD-STA Net, a neural framework integrating adaptive filtering and spatiotemporal dynamics learning for predicting high-dimensional chaotic systems governed by partial differential equations. The architecture combines: 1) An adaptive exponential smoothing module with position-aware decay coefficients for robust attractor reconstruction, 2) Parallel attention mechanisms capturing cross-temporal and spatial dependencies, 3) Dynamic gated fusion of multiscale features, and 4) Deep projection networks with dimension-scaling capabilities. Numerical experiments on nonlinear PDE systems demonstrate the model's effectiveness in maintaining prediction accuracy under both smooth and strongly chaotic regimes while exhibiting noise tolerance through adaptive filtering. Component ablation studies confirm critical contributions from each module, particularly highlighting the essential role of spatiotemporal attention in learning complex dynamical interactions. The framework shows promising potential for real-world applications requiring simultaneous handling of measurement uncertainties and high-dimensional nonlinear dynamics.

STFM: A Spatio-Temporal Information Fusion Model Based on Phase Space Reconstruction for Sea Surface Temperature Prediction

The sea surface temperature (SST), a key environmental parameter, is crucial to optimizing production planning, making its accurate prediction a vital research topic. However, the inherent nonlinearity of the marine dynamic system presents significant challenges. Current forecasting methods mainly include physics-based numerical simulations and data-driven machine learning approaches. The former, while describing SST evolution through differential equations, suffers from high computational complexity and limited applicability, whereas the latter, despite its computational benefits, requires large datasets and faces interpretability challenges. This study presents a prediction framework based solely on data-driven techniques. Using phase space reconstruction, we construct initial-delay attractor pairs with a mathematical homeomorphism and design a Spatio-Temporal Fusion Mapping (STFM) to uncover their intrinsic connections. Unlike conventional models, our method captures SST dynamics efficiently through phase space reconstruction and achieves high prediction accuracy with minimal training data in comparative tests

Global optimization of expensive black-box models based on asynchronous hybrid-criterion with interval reduction

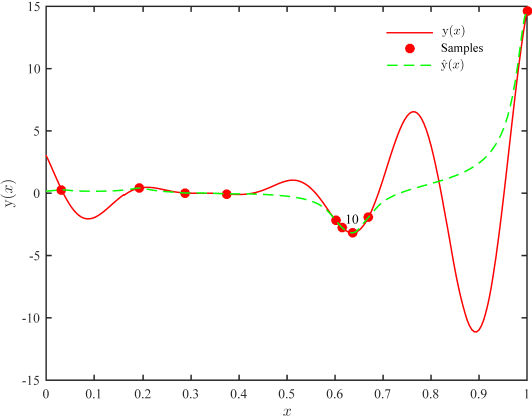
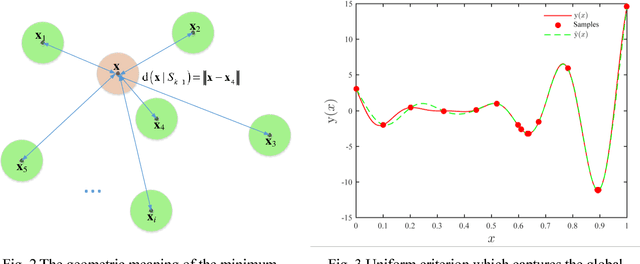
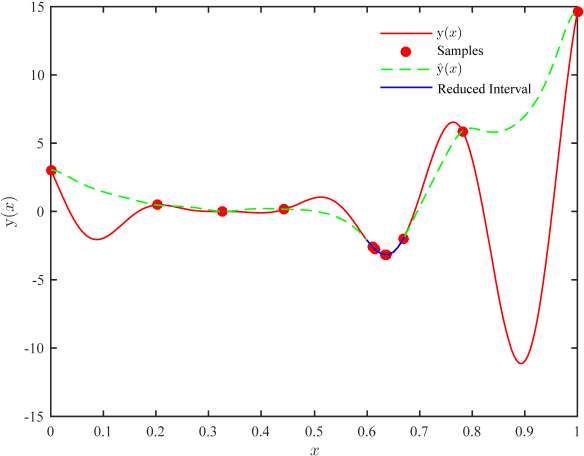
In this paper, a new sequential surrogate-based optimization (SSBO) algorithm is developed, which aims to improve the global search ability and local search efficiency for the global optimization of expensive black-box models. The proposed method involves three basic sub-criteria to infill new samples asynchronously to balance the global exploration and local exploitation. First, to capture the promising possible global optimal region, searching for the global optimum with genetic algorithm (GA) based on the current surrogate models of the objective and constraint functions. Second, to infill samples in the region with sparse samples to improve the global accuracy of the surrogate models, a grid searching with Latin hypercube sampling (LHS) with the current surrogate model is adopted to explore the sample space. Third, to accelerate the local searching efficiency, searching for a local optimum with sequential quadratic programming (SQP) based on the local surrogate models in the reduced interval, which involves some samples near the current optimum. When the new sample is too close to the existing ones, the new sample should be abandoned, due to the poor additional information. According to the three sub-criteria, the new samples are placed in the regions which have not been fully explored and includes the possible global optimum point. When a possible global optimum point is found, the local searching sub-criterion captures the local optimum around it rapidly. Numerical and engineering examples are used to verify the efficiency of the proposed method. The statistical results show that the proposed method has good global searching ability and efficiency.