A Factor Graph Approach to Scalable Multi-Output Gaussian Process Regression

Multi-output Gaussian process regression scales cubically in the number of observations times outputs, and dense kernel-matrix methods need bespoke handling whenever different outputs are observed at different inputs. We express multi-output Gaussian process regression as a Forney-style factor graph in which a nearest-neighbor chain orders a fixed candidate set of $C$ inputs into a one-dimensional sequence. Along this chain, latent Matérn processes evolve through linear-Gaussian transition factors, while the linear model of coregionalization mixes $L$ latent processes into $D$ outputs through a deterministic mixing factor and per-output scalar observation factors. Posterior computation reduces to exact Gaussian message passing on the chain at cost $\mathcal{O}(C(DL^2 + L^3))$ after chain construction, and missing observations omit their local factor without any covariance-matrix restructuring. The formulation therefore scales in the number of data samples and in the rate of missing observations, while remaining best suited to candidate sets in low input dimension.We compare the factor-graph formulation against an exact kernel-matrix baseline, a sparse-variational inducing-point baseline, and a nearest-neighbor baseline on a synthetic input-dimension sweep and on electricity time series forecasting. At low input dimension the factor-graph posterior tracks the exact kernel-matrix posterior closely, and the gap grows gradually as input dimension increases while staying competitive with both approximate baselines. On the electricity time series our factor-graph formulation matches all three baselines in forecast accuracy while scaling linearly in the number of data points, where the exact kernel-matrix method becomes infeasible and the inducing-point baseline remains substantially slower.

Expected Free Energy-based Planning as Variational Inference

We address the problem of planning under uncertainty, where an agent must choose actions that not only achieve desired outcomes but also reduce uncertainty. Traditional methods often treat exploration and exploitation as separate objectives, lacking a unified inferential foundation. Active inference, grounded in the Free Energy Principle, offers such a foundation by minimizing Expected Free Energy (EFE), a cost function that combines utility with epistemic drives like ambiguity resolution and novelty seeking. However, the computational burden of EFE minimization has remained a major obstacle to its scalability. In this paper, we show that EFE-based planning arises naturally from minimizing a variational free energy functional on a generative model augmented with preference and epistemic priors. This result reinforces theoretical consistency with the Free Energy Principle, by casting planning itself as variational inference. Our formulation yields optimal policies that jointly support goal achievement and information gain, while incorporating a complexity term that accounts for bounded computational resources. This unifying framework connects and extends existing methods, enabling scalable, resource-aware implementations of active inference agents.

Variational message passing for online polynomial NARMAX identification

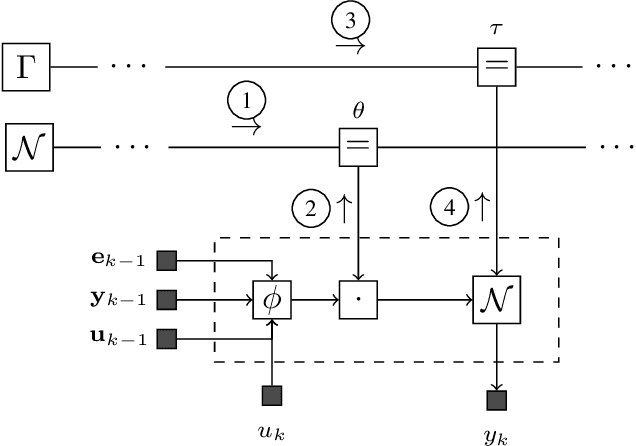
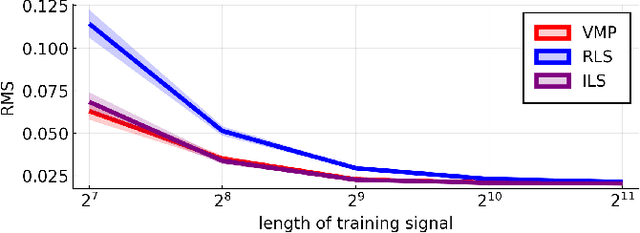
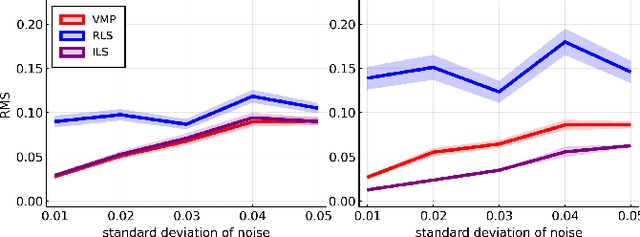
We propose a variational Bayesian inference procedure for online nonlinear system identification. For each output observation, a set of parameter posterior distributions is updated, which is then used to form a posterior predictive distribution for future outputs. We focus on the class of polynomial NARMAX models, which we cast into probabilistic form and represent in terms of a Forney-style factor graph. Inference in this graph is efficiently performed by a variational message passing algorithm. We show empirically that our variational Bayesian estimator outperforms an online recursive least-squares estimator, most notably in small sample size settings and low noise regimes, and performs on par with an iterative least-squares estimator trained offline.

AIDA: An Active Inference-based Design Agent for Audio Processing Algorithms

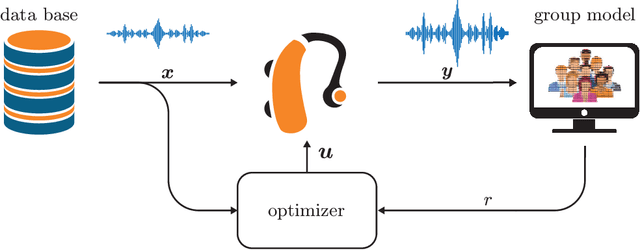
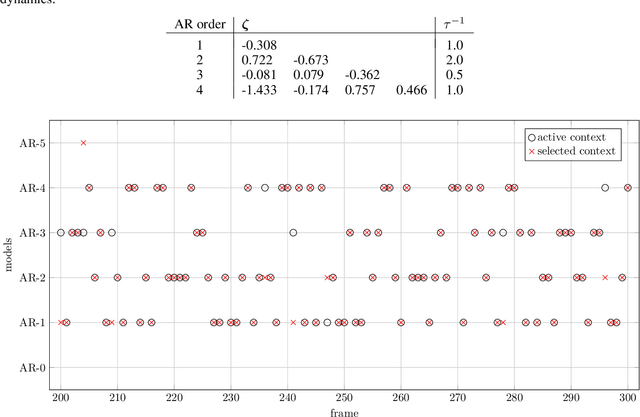
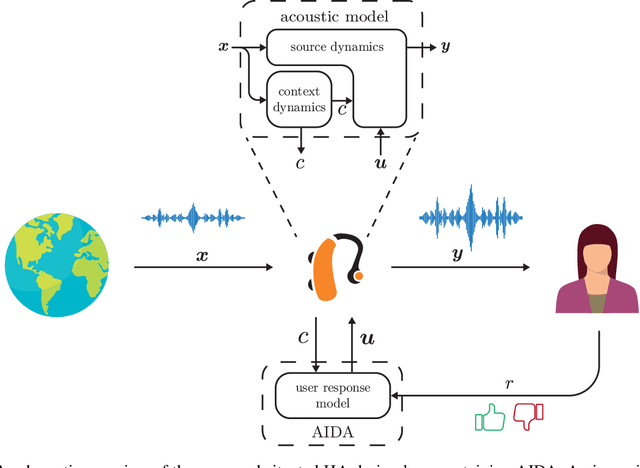
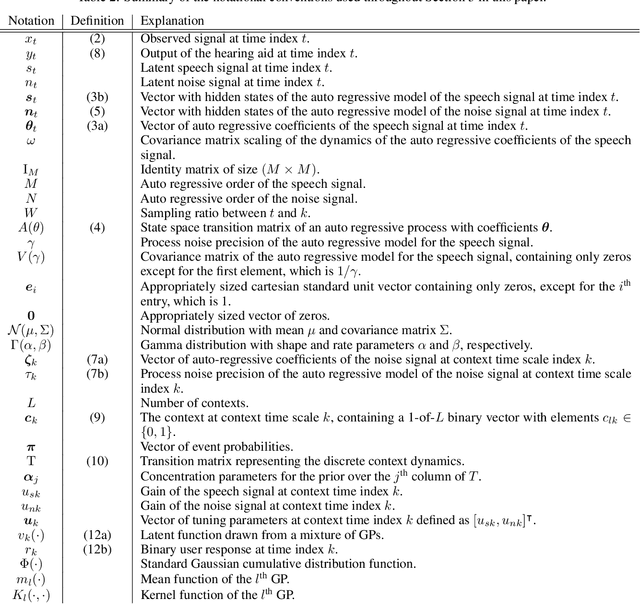
In this paper we present AIDA, which is an active inference-based agent that iteratively designs a personalized audio processing algorithm through situated interactions with a human client. The target application of AIDA is to propose on-the-spot the most interesting alternative values for the tuning parameters of a hearing aid (HA) algorithm, whenever a HA client is not satisfied with their HA performance. AIDA interprets searching for the "most interesting alternative" as an issue of optimal (acoustic) context-aware Bayesian trial design. In computational terms, AIDA is realized as an active inference-based agent with an Expected Free Energy criterion for trial design. This type of architecture is inspired by neuro-economic models on efficient (Bayesian) trial design in brains and implies that AIDA comprises generative probabilistic models for acoustic signals and user responses. We propose a novel generative model for acoustic signals as a sum of time-varying auto-regressive filters and a user response model based on a Gaussian Process Classifier. The full AIDA agent has been implemented in a factor graph for the generative model and all tasks (parameter learning, acoustic context classification, trial design, etc.) are realized by variational message passing on the factor graph. All verification and validation experiments and demonstrations are freely accessible at our GitHub repository.