Heuristic Algorithms for the Approximation of Mutual Coherence

Mutual coherence is a measure of similarity between two opinions. Although the notion comes from philosophy, it is essential for a wide range of technologies, e.g., the Wahl-O-Mat system. In Germany, this system helps voters to find candidates that are the closest to their political preferences. The exact computation of mutual coherence is highly time-consuming due to the iteration over all subsets of an opinion. Moreover, for every subset, an instance of the SAT model counting problem has to be solved which is known to be a hard problem in computer science. This work is the first study to accelerate this computation. We model the distribution of the so-called confirmation values as a mixture of three Gaussians and present efficient heuristics to estimate its model parameters. The mutual coherence is then approximated with the expected value of the distribution. Some of the presented algorithms are fully polynomial-time, others only require solving a small number of instances of the SAT model counting problem. The average squared error of our best algorithm lies below 0.0035 which is insignificant if the efficiency is taken into account. Furthermore, the accuracy is precise enough to be used in Wahl-O-Mat-like systems.

Can an NN model plainly learn planar layouts?

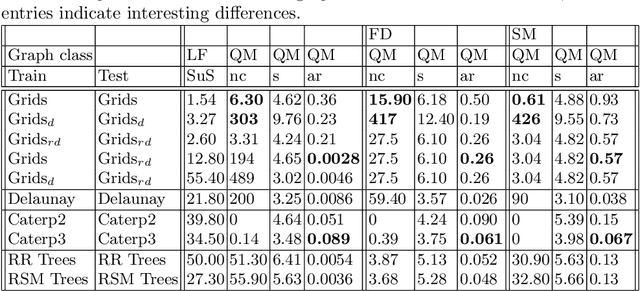
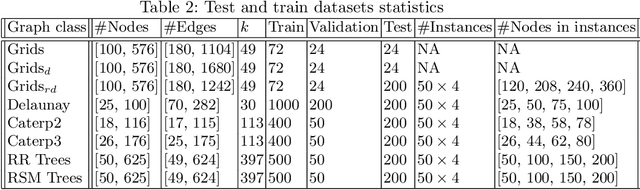
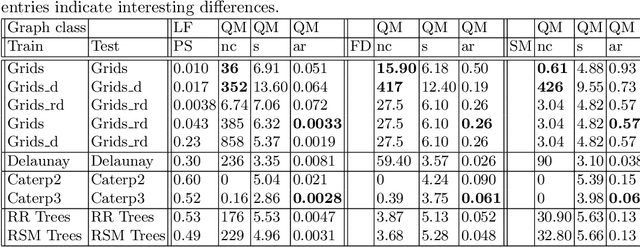
Planar graph drawings tend to be aesthetically pleasing. In this poster we explore a Neural Network's capability of learning various planar graph classes. Additionally, we also investigate the effectiveness of the model in generalizing beyond planarity. We find that the model can outperform conventional techniques for certain graph classes. The model, however, appears to be more susceptible to randomness in the data, and seems to be less robust than expected.

Aesthetic Discrimination of Graph Layouts

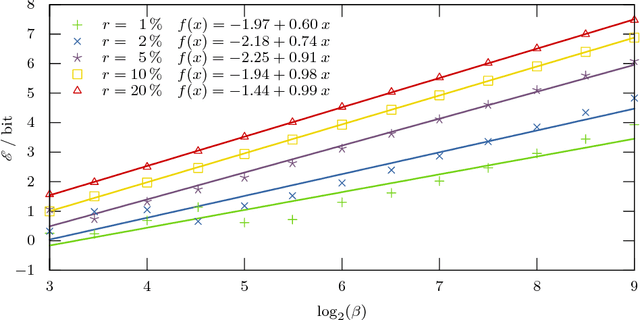
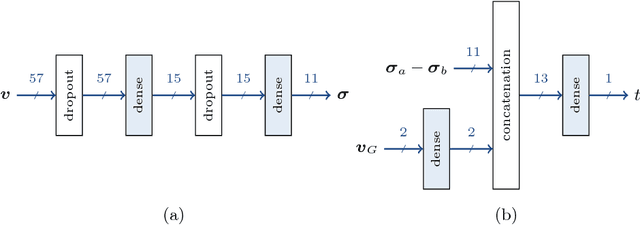
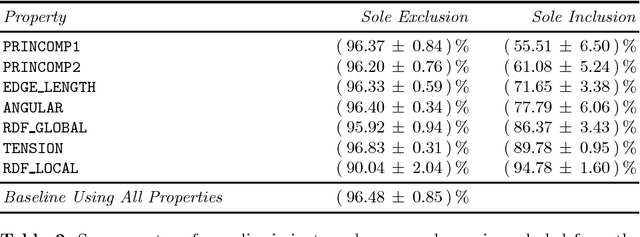
This paper addresses the following basic question: given two layouts of the same graph, which one is more aesthetically pleasing? We propose a neural network-based discriminator model trained on a labeled dataset that decides which of two layouts has a higher aesthetic quality. The feature vectors used as inputs to the model are based on known graph drawing quality metrics, classical statistics, information-theoretical quantities, and two-point statistics inspired by methods of condensed matter physics. The large corpus of layout pairs used for training and testing is constructed using force-directed drawing algorithms and the layouts that naturally stem from the process of graph generation. It is further extended using data augmentation techniques. The mean prediction accuracy of our model is 95.70%, outperforming discriminators based on stress and on the linear combination of popular quality metrics by a statistically significant margin.