Joint variable and rank selection for parsimonious estimation of high-dimensional matrices

We propose dimension reduction methods for sparse, high-dimensional multivariate response regression models. Both the number of responses and that of the predictors may exceed the sample size. Sometimes viewed as complementary, predictor selection and rank reduction are the most popular strategies for obtaining lower-dimensional approximations of the parameter matrix in such models. We show in this article that important gains in prediction accuracy can be obtained by considering them jointly. We motivate a new class of sparse multivariate regression models, in which the coefficient matrix has low rank and zero rows or can be well approximated by such a matrix. Next, we introduce estimators that are based on penalized least squares, with novel penalties that impose simultaneous row and rank restrictions on the coefficient matrix. We prove that these estimators indeed adapt to the unknown matrix sparsity and have fast rates of convergence. We support our theoretical results with an extensive simulation study and two data analyses.

SPADES and mixture models

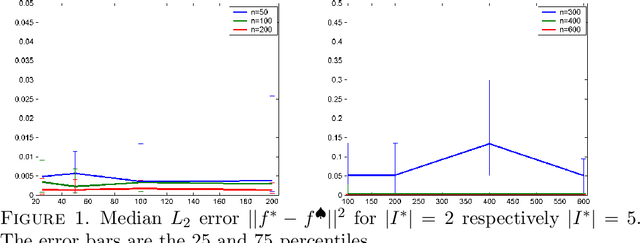
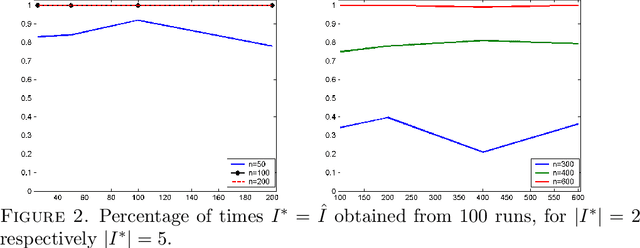
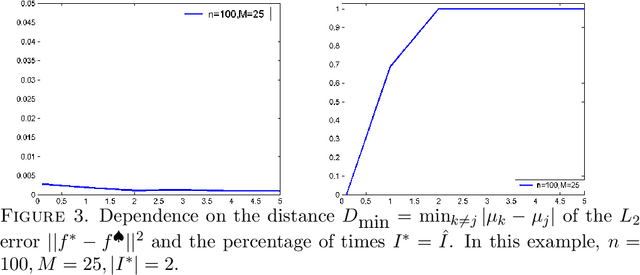
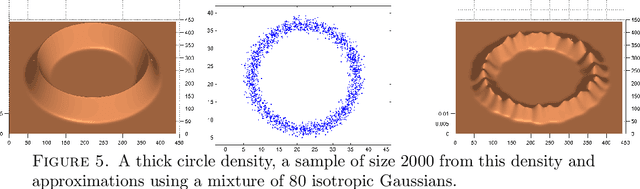
This paper studies sparse density estimation via $\ell_1$ penalization (SPADES). We focus on estimation in high-dimensional mixture models and nonparametric adaptive density estimation. We show, respectively, that SPADES can recover, with high probability, the unknown components of a mixture of probability densities and that it yields minimax adaptive density estimates. These results are based on a general sparsity oracle inequality that the SPADES estimates satisfy. We offer a data driven method for the choice of the tuning parameter used in the construction of SPADES. The method uses the generalized bisection method first introduced in \citebb09. The suggested procedure bypasses the need for a grid search and offers substantial computational savings. We complement our theoretical results with a simulation study that employs this method for approximations of one and two-dimensional densities with mixtures. The numerical results strongly support our theoretical findings.