Data-Assisted Vision-Based Hybrid Control for Robust Stabilization with Obstacle Avoidance via Learning of Perception Maps

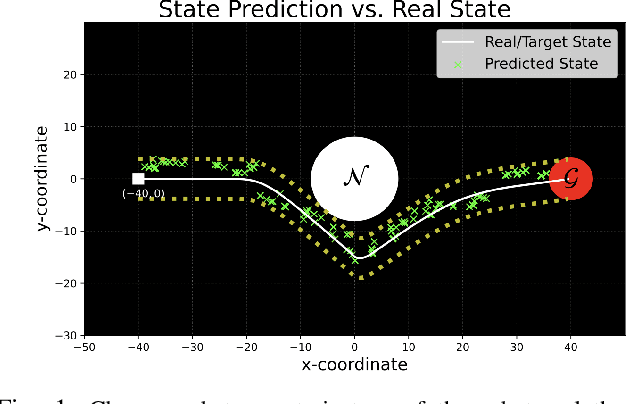
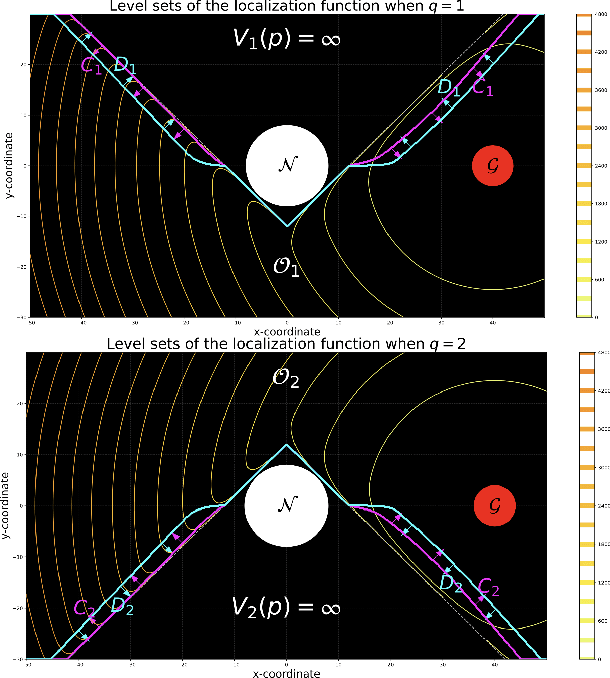
We study the problem of target stabilization with robust obstacle avoidance in robots and vehicles that have access only to vision-based sensors for the purpose of realtime localization. This problem is particularly challenging due to the topological obstructions induced by the obstacle, which preclude the existence of smooth feedback controllers able to achieve simultaneous stabilization and robust obstacle avoidance. To overcome this issue, we develop a vision-based hybrid controller that switches between two different feedback laws depending on the current position of the vehicle using a hysteresis mechanism and a data-assisted supervisor. The main innovation of the paper is the incorporation of suitable perception maps into the hybrid controller. These maps can be learned from data obtained from cameras in the vehicles and trained via convolutional neural networks (CNN). Under suitable assumptions on this perception map, we establish theoretical guarantees for the trajectories of the vehicle in terms of convergence and obstacle avoidance. Moreover, the proposed vision-based hybrid controller is numerically tested under different scenarios, including noisy data, sensors with failures, and cameras with occlusions.

Accelerated Continuous-Time Approximate Dynamic Programming via Data-Assisted Hybrid Control

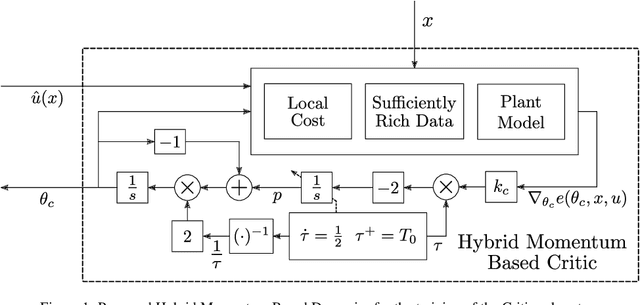
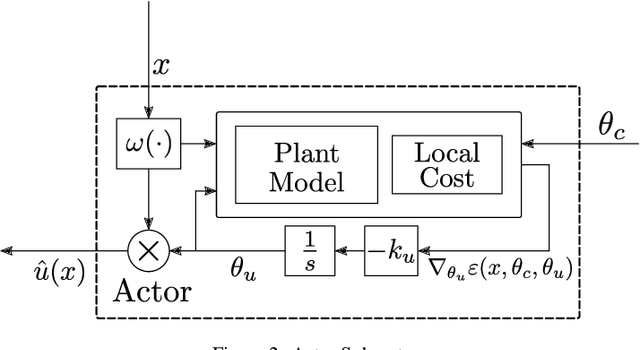
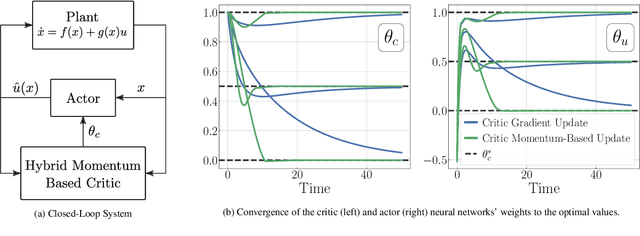
We introduce a new closed-loop architecture for the online solution of approximate optimal control problems in the context of continuous-time systems. Specifically, we introduce the first algorithm that incorporates dynamic momentum in actor-critic structures to control continuous-time dynamic plants with an affine structure in the input. By incorporating dynamic momentum in our algorithm, we are able to accelerate the convergence properties of the closed-loop system, achieving superior transient performance compared to traditional gradient-descent based techniques. In addition, by leveraging the existence of past recorded data with sufficiently rich information properties, we dispense with the persistence of excitation condition traditionally imposed on the regressors of the critic and the actor. Given that our continuous-time momentum-based dynamics also incorporate periodic discrete-time resets that emulate restarting techniques used in the machine learning literature, we leverage tools from hybrid dynamical systems theory to establish asymptotic stability properties for the closed-loop system. We illustrate our results with a numerical example.