Get our free extension to see links to code for papers anywhere online!Free add-on: code for papers everywhere!Free add-on: See code for papers anywhere!
Carl Johnell
Efficient Optimization of Dominant Set Clustering with Frank-Wolfe Algorithms
Aug 05, 2020Figures and Tables: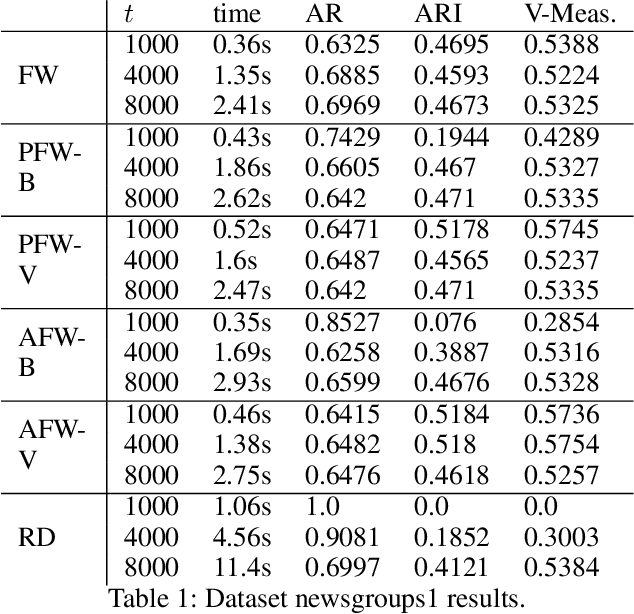
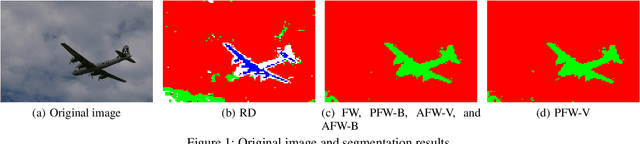
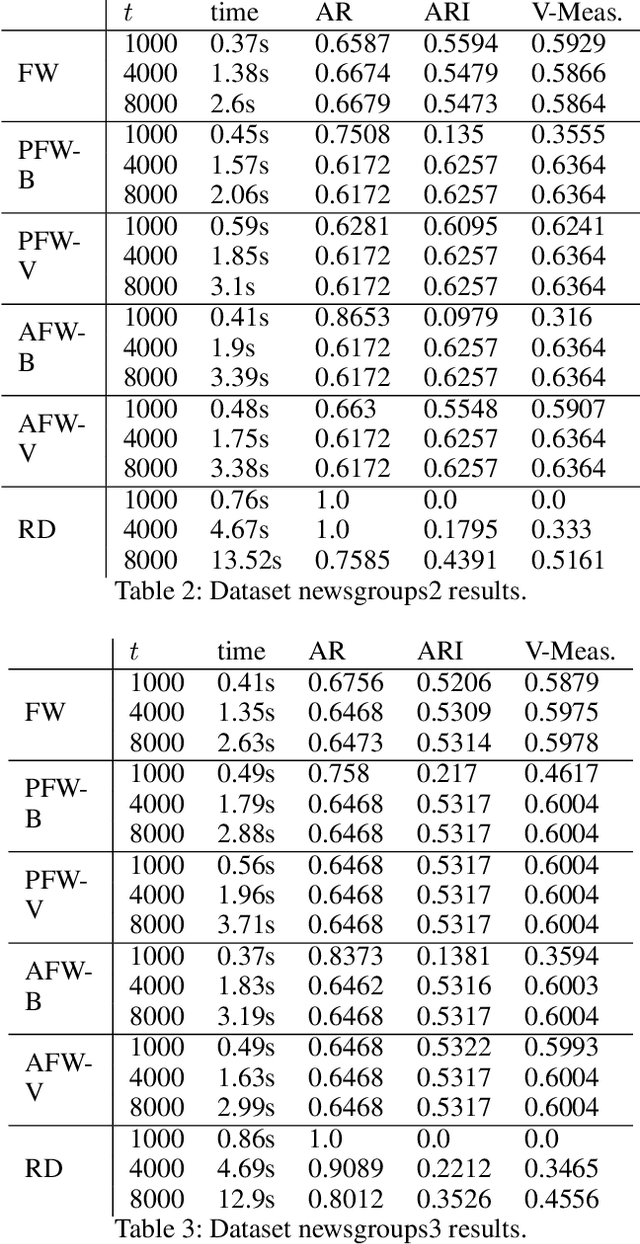
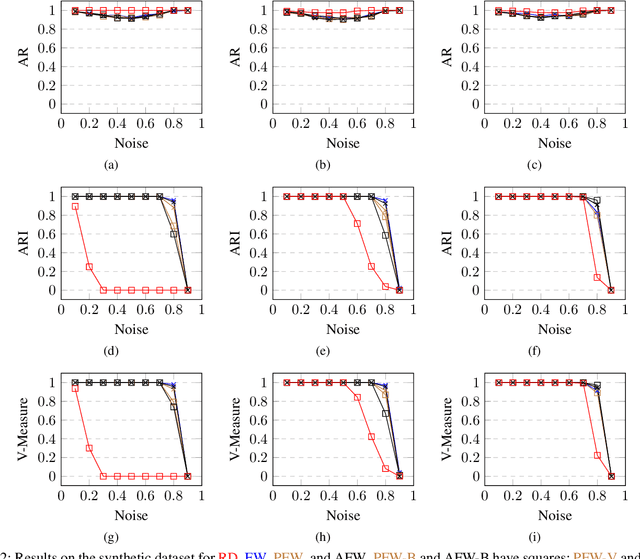




Abstract:We study Frank-Wolfe algorithms -- standard, pairwise, and away-steps -- for efficient optimization of Dominant Set Clustering. We present a unified and computationally efficient framework to employ the different variants of Frank-Wolfe methods, and we investigate its effectiveness via several experimental studies. In addition, we provide explicit convergence rates for the algorithms in terms of the so-called Frank-Wolfe gap. The theoretical analysis has been specialized to the problem of Dominant Set Clustering and is thus more easily accessible compared to prior work.
Via
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge