Can a Fruit Fly Learn Word Embeddings?

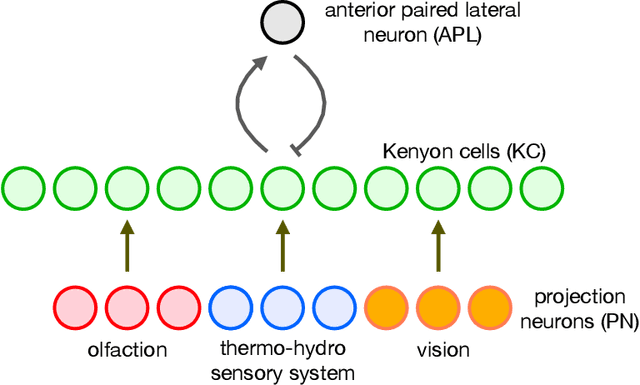
The mushroom body of the fruit fly brain is one of the best studied systems in neuroscience. At its core it consists of a population of Kenyon cells, which receive inputs from multiple sensory modalities. These cells are inhibited by the anterior paired lateral neuron, thus creating a sparse high dimensional representation of the inputs. In this work we study a mathematical formalization of this network motif and apply it to learning the correlational structure between words and their context in a corpus of unstructured text, a common natural language processing (NLP) task. We show that this network can learn semantic representations of words and can generate both static and context-dependent word embeddings. Unlike conventional methods (e.g., BERT, GloVe) that use dense representations for word embedding, our algorithm encodes semantic meaning of words and their context in the form of sparse binary hash codes. The quality of the learned representations is evaluated on word similarity analysis, word-sense disambiguation, and document classification. It is shown that not only can the fruit fly network motif achieve performance comparable to existing methods in NLP, but, additionally, it uses only a fraction of the computational resources (shorter training time and smaller memory footprint).

Large Associative Memory Problem in Neurobiology and Machine Learning

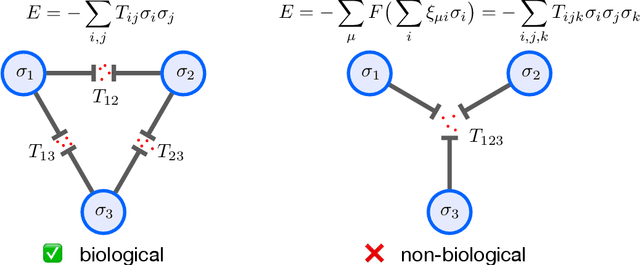
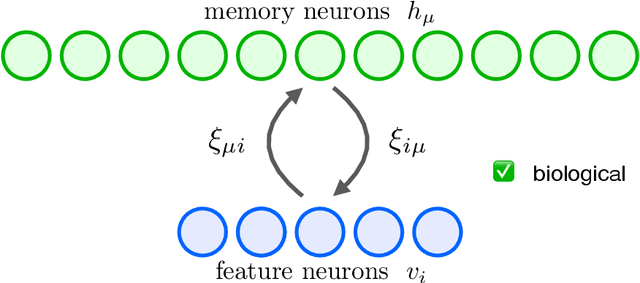
Dense Associative Memories or modern Hopfield networks permit storage and reliable retrieval of an exponentially large (in the dimension of feature space) number of memories. At the same time, their naive implementation is non-biological, since it seemingly requires the existence of many-body synaptic junctions between the neurons. We show that these models are effective descriptions of a more microscopic (written in terms of biological degrees of freedom) theory that has additional (hidden) neurons and only requires two-body interactions between them. For this reason our proposed microscopic theory is a valid model of large associative memory with a degree of biological plausibility. The dynamics of our network and its reduced dimensional equivalent both minimize energy (Lyapunov) functions. When certain dynamical variables (hidden neurons) are integrated out from our microscopic theory, one can recover many of the models that were previously discussed in the literature, e.g. the model presented in ''Hopfield Networks is All You Need'' paper. We also provide an alternative derivation of the energy function and the update rule proposed in the aforementioned paper and clarify the relationships between various models of this class.

Bio-Inspired Hashing for Unsupervised Similarity Search

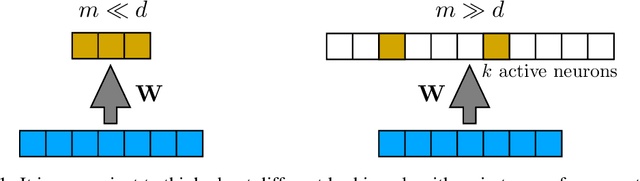
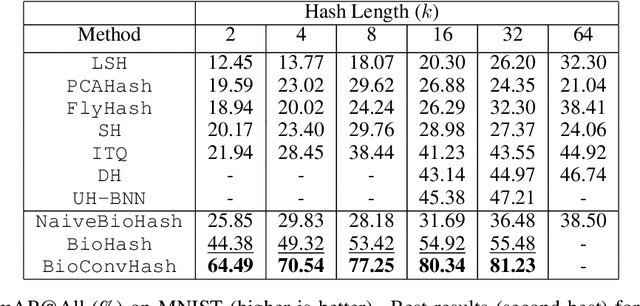
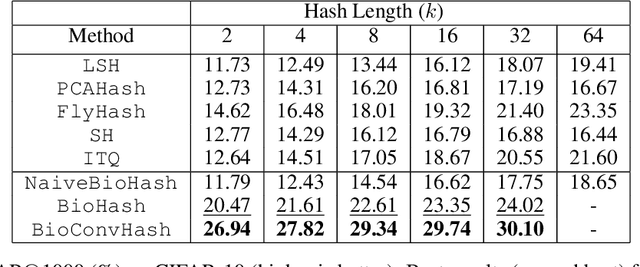
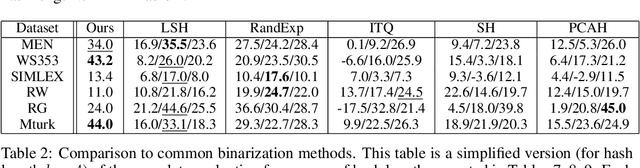
The fruit fly Drosophila's olfactory circuit has inspired a new locality sensitive hashing (LSH) algorithm, FlyHash. In contrast with classical LSH algorithms that produce low dimensional hash codes, FlyHash produces sparse high-dimensional hash codes and has also been shown to have superior empirical performance compared to classical LSH algorithms in similarity search. However, FlyHash uses random projections and cannot learn from data. Building on inspiration from FlyHash and the ubiquity of sparse expansive representations in neurobiology, our work proposes a novel hashing algorithm BioHash that produces sparse high dimensional hash codes in a data-driven manner. We show that BioHash outperforms previously published benchmarks for various hashing methods. Since our learning algorithm is based on a local and biologically plausible synaptic plasticity rule, our work provides evidence for the proposal that LSH might be a computational reason for the abundance of sparse expansive motifs in a variety of biological systems. We also propose a convolutional variant BioConvHash that further improves performance. From the perspective of computer science, BioHash and BioConvHash are fast, scalable and yield compressed binary representations that are useful for similarity search.

Local Unsupervised Learning for Image Analysis

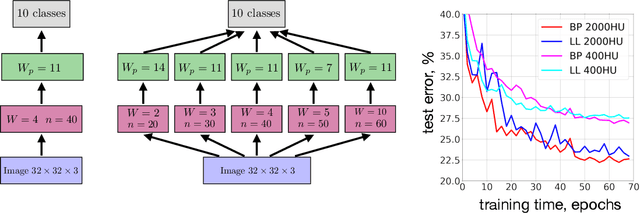
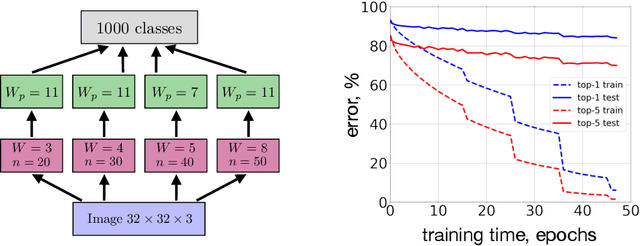
Local Hebbian learning is believed to be inferior in performance to end-to-end training using a backpropagation algorithm. We question this popular belief by designing a local algorithm that can learn convolutional filters at scale on large image datasets. These filters combined with patch normalization and very steep non-linearities result in a good classification accuracy for shallow networks trained locally, as opposed to end-to-end. The filters learned by our algorithm contain both orientation selective units and unoriented color units, resembling the responses of pyramidal neurons located in the cytochrome oxidase 'interblob' and 'blob' regions in the primary visual cortex of primates. It is shown that convolutional networks with patch normalization significantly outperform standard convolutional networks on the task of recovering the original classes when shadows are superimposed on top of standard CIFAR-10 images. Patch normalization approximates the retinal adaptation to the mean light intensity, important for human vision. We also demonstrate a successful transfer of learned representations between CIFAR-10 and ImageNet 32x32 datasets. All these results taken together hint at the possibility that local unsupervised training might be a powerful tool for learning general representations (without specifying the task) directly from unlabeled data.

Unsupervised Learning by Competing Hidden Units

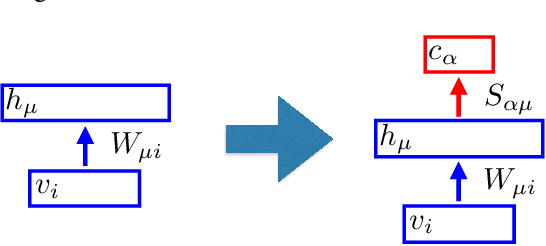
It is widely believed that the backpropagation algorithm is essential for learning good feature detectors in early layers of artificial neural networks, so that these detectors are useful for the task performed by the higher layers of that neural network. At the same time, the traditional form of backpropagation is biologically implausible. In the present paper we propose an unusual learning rule, which has a degree of biological plausibility, and which is motivated by Hebb's idea that change of the synapse strength should be local - i.e. should depend only on the activities of the pre and post synaptic neurons. We design a learning algorithm that utilizes global inhibition in the hidden layer, and is capable of learning early feature detectors in a completely unsupervised way. These learned lower layer feature detectors can be used to train higher layer weights in a usual supervised way so that the performance of the full network is comparable to the performance of standard feedforward networks trained end-to-end with a backpropagation algorithm.

Dense Associative Memory is Robust to Adversarial Inputs

Deep neural networks (DNN) trained in a supervised way suffer from two known problems. First, the minima of the objective function used in learning correspond to data points (also known as rubbish examples or fooling images) that lack semantic similarity with the training data. Second, a clean input can be changed by a small, and often imperceptible for human vision, perturbation, so that the resulting deformed input is misclassified by the network. These findings emphasize the differences between the ways DNN and humans classify patterns, and raise a question of designing learning algorithms that more accurately mimic human perception compared to the existing methods. Our paper examines these questions within the framework of Dense Associative Memory (DAM) models. These models are defined by the energy function, with higher order (higher than quadratic) interactions between the neurons. We show that in the limit when the power of the interaction vertex in the energy function is sufficiently large, these models have the following three properties. First, the minima of the objective function are free from rubbish images, so that each minimum is a semantically meaningful pattern. Second, artificial patterns poised precisely at the decision boundary look ambiguous to human subjects and share aspects of both classes that are separated by that decision boundary. Third, adversarial images constructed by models with small power of the interaction vertex, which are equivalent to DNN with rectified linear units (ReLU), fail to transfer to and fool the models with higher order interactions. This opens up a possibility to use higher order models for detecting and stopping malicious adversarial attacks. The presented results suggest that DAM with higher order energy functions are closer to human visual perception than DNN with ReLUs.

Dense Associative Memory for Pattern Recognition

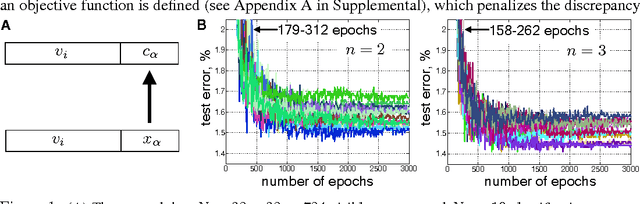
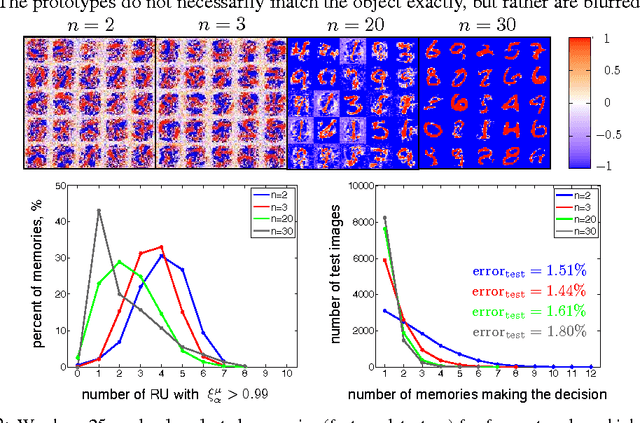
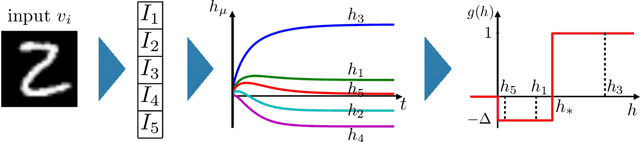
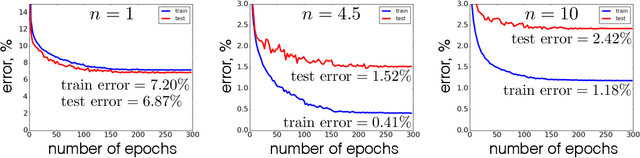
A model of associative memory is studied, which stores and reliably retrieves many more patterns than the number of neurons in the network. We propose a simple duality between this dense associative memory and neural networks commonly used in deep learning. On the associative memory side of this duality, a family of models that smoothly interpolates between two limiting cases can be constructed. One limit is referred to as the feature-matching mode of pattern recognition, and the other one as the prototype regime. On the deep learning side of the duality, this family corresponds to feedforward neural networks with one hidden layer and various activation functions, which transmit the activities of the visible neurons to the hidden layer. This family of activation functions includes logistics, rectified linear units, and rectified polynomials of higher degrees. The proposed duality makes it possible to apply energy-based intuition from associative memory to analyze computational properties of neural networks with unusual activation functions - the higher rectified polynomials which until now have not been used in deep learning. The utility of the dense memories is illustrated for two test cases: the logical gate XOR and the recognition of handwritten digits from the MNIST data set.